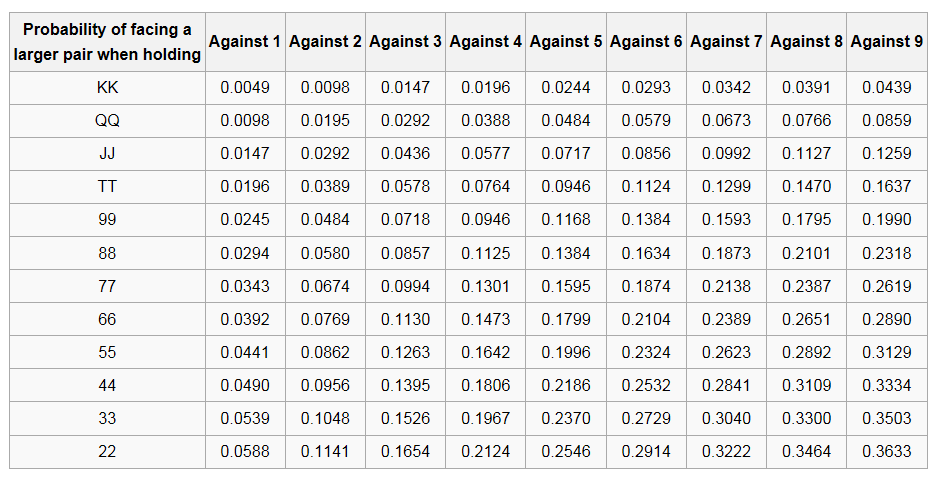
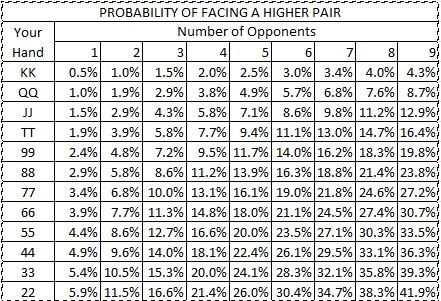
My approach would be to start with the case of holding KK against one player, where the chance of them holding a higher pair (in this case, exactly AA) is:
(4/50)*(3/49)
or
(4*3)/(50*49)
if you'd prefer. This comes to 0.0049 or 0.49%.
From here, we can extrapolate for the number of players by simply multiplying the above by the number of players, so against 2 players, we are twice as likely to face AA (this ignore card removal, see below), and so we expect this 0.0049 * 2 = 0.0098 or 0.98% of the time.
Similarly, we can extrapolate our chances of facing a larger pair when we ourselves hold a smaller pair by just multiplying by the number of larger pairs. When we hold QQ, the chance of a single opponent holding a higher pair is twice as large (as holding AA or KK are mutually exclusive). We can show this explicitly:
[chance of facing AA or KK when holding QQ]
= (8/50)*(3/49)
= 0.0098 (0.98%)
or by simply doubling our original result.
By this method, we can calculate all of the values:
/---------------------------------------------------------------------------------------\
| | Opponents |
| Hand | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|------+--------+--------+--------+--------+--------+--------+--------+--------+--------|
| KK | 0.49% | 0.98% | 1.47% | 1.96% | 2.45% | 2.94% | 3.43% | 3.92% | 4.41% |
| QQ | 0.98% | 1.96% | 2.94% | 3.92% | 4.90% | 5.88% | 6.86% | 7.84% | 8.82% |
| JJ | 1.47% | 2.94% | 4.41% | 5.88% | 7.35% | 8.82% | 10.29% | 11.76% | 13.22% |
| TT | 1.96% | 3.92% | 5.88% | 7.84% | 9.80% | 11.76% | 13.71% | 15.67% | 17.63% |
| 99 | 2.45% | 4.90% | 7.35% | 9.80% | 12.24% | 14.69% | 17.14% | 19.59% | 22.04% |
| 88 | 2.94% | 5.88% | 8.82% | 11.76% | 14.69% | 17.63% | 20.57% | 23.51% | 26.45% |
| 77 | 3.43% | 6.86% | 10.29% | 13.71% | 17.14% | 20.57% | 24.00% | 27.43% | 30.86% |
| 66 | 3.92% | 7.84% | 11.76% | 15.67% | 19.59% | 23.51% | 27.43% | 31.35% | 35.27% |
| 55 | 4.41% | 8.82% | 13.22% | 17.63% | 22.04% | 26.45% | 30.86% | 35.27% | 39.67% |
| 44 | 4.90% | 9.80% | 14.69% | 19.59% | 24.49% | 29.39% | 34.29% | 39.18% | 44.08% |
| 33 | 5.39% | 10.78% | 16.16% | 21.55% | 26.94% | 32.33% | 37.71% | 43.10% | 48.49% |
| 22 | 5.88% | 11.76% | 17.63% | 23.51% | 29.39% | 35.27% | 41.14% | 47.02% | 52.90% |
\---------------------------------------------------------------------------------------/
However, this is not strictly the correct set of values, because the chances of a player making a pair are not mutually exclusive from the chances of another player in the same hand making a pair.
Consider for example a game with 9 players - the chances of a single player getting a pair (without any other information) is 3/52 = 0.0577 (5.77%), but what if we already know that some players already have pairs? Let's say for example that we know the hands of 3 other players at the table, who each have 44, 77 and KK respectively. Well now our chances of making a pair are:
[chance of making 44, 77 or KK] + [chance of making any other pair]
= [(6/46)*(1/45)] + [(40/46)*(3/45)]
= 0.0609 (6.09%)
We have a greater chance of making a pair because other players have already made pairs, which has polarised the deck with regards to pairs. Taking this to its logical conclusion, think about a situation at a 10-handed table where all 9 of your opponents have pairs (AA x 2, KK x 2, QQ x 2, JJ x 2 and TT). Now our chances of making a pair are:
[chance of making JJ+] + [chance of making TT] + [chance of making 22-99]
= [0] + [(2/34)*(1/33)] + [(32/34)*(3/33)]
= 0.0873 (8.73%)
Similarly, sometimes if we know that other players have not made pairs, our chances of making pairs also increases; for example, if we know 3 players hold AK, QJ and T9 respectively, our chances of making a pair are:
[chance of making 99+] + [chance of making 22-99]
= [(18/46)*(2/45)] + [(32/46)*(3/45)]
= 0.0638 (6.38%)
However, if lots of different ranks are known to be in other players' hands, our chances to make a pair will be decreased. For example, if we are in a 10-handed game where we know 6 of our opponents hold AK, QJ, T9, 87, 65, 43, our chances of making a pair are:
[chance of making 22] + [chance of making 33+]
= [(4/40)*(3/39)] + [(36/40)*(2/39)]
= 0.0538 (5.38%)
For this reason, my simplified approach above is not 100% accurate, because it is not correct to say that if the chance of facing an overpair with KK against one opponent is 0.49%, then it must be double this chance against two opponents - this is flawed because the chance of that second player having a pair is affected by what the first player held. At least that's my understanding.
In any case, the numbers above will certainly be close enough to the "real" figures to be useful to you, and hopefully this answer has helped show you how these numbers are calculated.